(Each Question Carries 10 Marks)
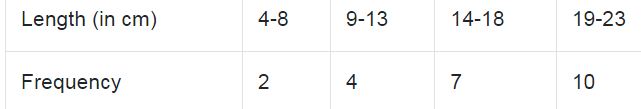
- Find the mean and standard deviation of the data from the following table:
- In a group of 20 adults, 4 out of 7 women and 3 out of 13 men wear glasses. What is
(i) the probability that a person chosen at random from the group is a woman or someone who wears glasses; and
(ii) Given a person randomly chosen from the woman group, what is the probability that she wears glasses.
- Given the following statistics, what is the probability that a woman over 50 has breast cancer if she has a positive mammogram result?
- One percent of women over 50 have breast cancer.
- Ninety percent of women over 50 who have breast cancer test positive
on mammograms.
- Eight percent of women over 50 who do not have breast cancer test positive on mammograms.
- The average number of oil tankers arriving each day at a certain port is 3. For the next seven days, the port can only handle 3 tankers per day due to some urgent repair works to be carried there. What is the probability that on a given days during this period, tankers have to be turned away?
- (i) The scores of a Mathematics Olympiad Test are normally distributed with mean 50 and standard deviation 10, find the probability that the score of a randomly chosen participant has a test score between 50 and 80.
(ii) The probability of a certain kind of component will survive a given shock test is ¾. Find the probability that exactly 2 of the 4 components tested will survive.
- The average mark for an exam is 74 with standard deviation = 7. All the marks are integer values between 0 and 100 inclusively. If the highest 8.08% of the marks are to be awarded with the highest-grade A and marks are curved to follow a normal distribution, what is the lowest possible mark for A and the highest possible for the next lower grade B?
Suppose that against a certain opponent the number of points the MIT basketball team scores is normally distributed with unknown mean and unknown variance
- Suppose that over the course of the last 10 games between the two teams MIT scored the following points:
59, 62, 59, 74, 70, 61, 62, 66, 62, 75 Compute a 95% confidence interval for 𝜃.
- The length of life of light bulbs manufactured by an electrical firm is normally distributed with mean 800 hours and standard deviation 40 hours. What is the probability that a random batch of 16 of these light bulbs bought will have an average life of less than 775 hours.
- A drug company is testing a drug intended to increase heart rate. A sample of 100 yielded a mean increase of 1.4 beats per minute, with a population standard deviation known to be 3.6. T e s t t h e c l a i m t h a t t h e d r u g i n c r e a s e s t h e h e a r t r a t e a t 0.025 level of significance.
- The data below show the sugar content of a fruit (SUGAR) for different numbers of days after picking (DAYS).

Draw the scatterplot and obtain the least square regression line to predict sugar content based on the number of days after picking.


